Character table for point group C7

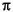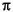=exp(2i/7)
C7 E C7 (C7)2 (C7)3 (C7)4 (C7)5 (C7)6
linear functions,
rotations
quadratic
functions
cubic
functions
A +1 +1 +1 +1 +1 +1 +1 z, Rz x2+y2, z2 z3, z(x2+y2)
E1 +1
+1		 +
+*		+2
+2*		+3
+3*		+3*
+3		+2*
+2		+*
+		x+iy, Rx+iRy
x-iy, Rx-iRy		 (xz, yz) (xz2, yz2) [x(x2+y2), y(x2+y2)]
E2 +1
+1		 +2
+2*		+3*
+3		+*
+		+
+*		+3
+3*		+2*
+2		- (x2-y2, xy) [xyz, z(x2-y2)]
E3 +1
+1		 +3
+3*		+*
+		+2
+2*		+2*
+2		+
+*		+3*
+3		- - [y(3x2-y2), x(x2-3y2)]


Additional information

Number of symmetry elements h = 7
Number of irreducible representations n = 7
Number of real irreducible representations n = 4
Abelian group yes
Optical Isomerism (Chirality) yes
Polar yes


Reduction formula for point group C7

Type of representation

Information for point groups with complex irreducible representations

general 3N vib

E C7 (C7)2 (C7)3 (C7)4 (C7)5 (C7)6



Multipoles

dipole (p) A+E1
quadrupole (d) A+E1+E2
octopole (f) A+E1+E2+E3
hexadecapole (g) A+E1+E2+2E3
32-pole (h) A+E1+2E2+2E3
64-pole (i) A+2E1+2E2+2E3
128-pole (j) 3A+2E1+2E2+2E3
256-pole(k) 3A+3E1+2E2+2E3
512-pole (l) 3A+3E1+3E2+2E3

First nonvanishing multipole: dipole

Literature


Character tables for chemically important point groups Computational Laboratory for Analysis, Modeling and Visualization Constructor University Bremen
Last update November, 13th 2023 by A. Gelessus, Impressum, Datenschutzerklärung/DataPrivacyStatement